Display Technology Shoot-Out

Comparing CRT, LCD, Plasma and DLP Displays

 

Dr. Raymond M. Soneira

President, DisplayMate Technologies Corp.

Copyright © 1990-2005 by DisplayMate Technologies Corporation. All Rights Reserved.
This article, or any part thereof, may not be copied, reproduced, mirrored, distributed or incorporated
into any other work without the prior written permission of DisplayMate Technologies Corporation

 

Part II  –  Gray-Scale and Color Accuracy

 

Article Links:  Overview  Part I  Part II  Part III  Part IV

Printing: If your browser is improperly printing some pages with text cutoff

 on the right edge then either print in Landscape mode or reduce the font

size (View Menu - Text Size) and margins (File Menu - Page Setup).

 

 

Introduction

This is Part II of a four part article series describing an in-depth comparison between CRT, LCD, Plasma and DLP display technologies in order to analyze the relative strengths and weaknesses of each. In Part I we measured, analyzed and compared primary specs like Black-Level, Color Temperature, Peak Brightness, Dynamic Range, and Display Contrast for each display technology. Click this link to read Part I. Here in Part II we continue with Gray-Scale, Gamma, Primary Chromaticities and Color Gamut to see how they all affect color and gray-scale accuracy.

 

We chose the top performer for each display technology using our own DisplayMate Best Video Hardware Guide. The candidates included a 40” direct-view LCD (NEC LCD4000), a 61” Plasma (NEC 61XM2), a 50” DLP Rear Projection (Optoma RD-50), and a much smaller CRT 19” professional High Definition studio monitor (Sony PVM-20L5), which was used as the reference standard for color and gray-scale accuracy. It’s important to emphasize that this article is designed as a comparison of four different display technologies and not as an editorial review of the above models. By comparing a top performing model in each technology we are in effect examining the state-of-the-art for that technology. We will be looking at fundamental image and picture quality performance issues and not the implementation details and idiosyncrasies of any particular model.

 

How We Tested:

The central concept for this article was to carefully set up, test and evaluate all of the display technologies at the same time under identical conditions and procedures, using advanced instrumentation where appropriate. All of the displays were set up side-by-side for simultaneous comparative viewing in a completely dark lab. The simultaneous viewing allowed us to detect subtle differences between the displays. We used computer and video-based test patterns, plus DVD, television, and computer applications, including a wide selection of test patterns from our own DisplayMate for Windows Multimedia Edition (see www.displaymate.com). All of the photometry and colorimetry measurements were made with a Konica Minolta CS-1000, which is a high-end laboratory Spectroradiometer with a narrow 1 degree acceptance angle for light emitted by the display. This advanced instrument was crucial for making precise comparisons between the different display technologies. See Part I for an in-depth discussion of the test methods and instrumentation that were used.

 

Gray-Scale

In Part I we measured the extremes of display brightness: the black-level and peak intensity white. Here we’re going to carefully examine all of the intensities in between, which is referred to as the display’s Gray-Scale (technically the Transfer Function). This is the signature of a display; it’s what gives the display its own unique look and performance characteristics. While each display technology has its own native gray-scale, known as the Transfer Characteristic of the display device, signal processing electronics within the display modifies this to produce the gray-scale that we actually see (and measure). There are two reasons why this is necessary. First, the native gray-scale for most display technologies is either unsuitable or sub-optimal for accurate image reproduction. Second, we need to have a standard gray-scale so that images will be accurately reproduced on any display or display technology. The accepted standard is the CRT’s own native gray-scale. There are two reasons why the CRT is the standard: first, it was until recently the only prevalent display technology so new technologies had to mimic its behavior if they were to be accepted; second, it turns out that the CRT’s native gray-scale is actually very close to the theoretical ideal (see below). We are incredibly lucky that the CRT came first and has served us well as an imaging device for over 75 years.

 

It turns out that the term Gray-Scale is an unfortunate word choice because this same relation actually describes the brightness scale for all colors, not just the grays, which are shades of white (see Figure 2, below). That’s because the same functional form must apply to each of the red, green and blue display primaries in order that the display’s color balance not vary with brightness. Since all colors are mixtures of the primaries, the same brightness relation applies to all colors not just gray/white. (Each color has its own unique peak brightness value but the brightness variations are all identical.) As we’ll see shortly, the shape of the Gray-Scale has a major impact on not only image brightness and contrast, but also on hue and color saturation.

 

Before going any further we first need to define exactly what is meant by the Gray-Scale. It is the brightness or amount of visible light (photometrically the Luminance, see Part I) that a display produces for a given level of input signal. (This applies for every pixel in the image.) For example, a maximum signal produces peak white and a zero signal produces the closest approximation to black that the display can provide. We measured the gray-scale using a set of our own DisplayMate for Windows test patterns together with a Konica Minolta CS-1000 Spectroradiometer. As we increase the signal from zero to maximum the display brightness also increases in a particular way that we can measure and then plot on a graph. This graph of brightness (luminance) versus signal intensity level is called the display’s Gray-Scale. The input signal can be specified in many different but equivalent ways. For computers it’s generally on a scale of 0 to 255, with 0 for black and 255 for peak white. For most digital video it’s 16 for black to 235 for peak white and for analog video it’s generally specified in IRE units, from either 0 or 7.5 for black to 100 for peak white. To simplify matters we’ll describe the input signal intensity level as a scale going from 0 percent for black to 100 percent for peak white. The brightness scale will be luminance in cd/m² (candelas per square meter), the same as in Part I. Note that we will be informally referring to Luminance as brightness and will use the two terms interchangeably throughout the article.

 

 

Gamma

Gamma is a popular, yet widely misunderstood number that describes the steepness of a display’s gray-scale as it increases from black to peak white. The gray-scale is not linear as most people presume, but instead logarithmic (mathematically it’s actually called a power-law, which behaves linearly on logarithmic scales, see below) because that’s how standard CRTs behave, and also because that corresponds well with the eye’s own logarithmic response (which is also a power-law). This complementary power-law behavior is one reason why the CRT has performed so well as a display technology.

 

While you normally see the gray-scale plotted as a linear graph, that’s really not the proper graph to use. Here’s why: what matters to the eye are ratios of brightness not differences in brightness. When comparing two intensities or mixing two colors it is their brightness ratio that determines what your eye sees. (Ratios are just divisions and differences are just subtractions between any two values.) A linear graph shows differences uniformly, which is not what we want. Since the eye responds to brightness ratios we need a graph that displays ratios uniformly. That’s just what a logarithmic graph does. You don’t need any advanced math to understand logarithmic graphs, just pay attention to the scale values. They’re arranged so that any given ratio corresponds to a fixed distance anywhere along the scale. For example, the distances between 80, 40, 20 and 10 (on the horizontal axis in Figures 1 and 2 below) are all the same because each is a 2:1 ratio.

 

Measurements

Below are logarithmic plots of the gray-scales as measured with the Konica Minolta CS-1000 Spectroradiometer and a set of DisplayMate for Windows test patterns. Both scales are logarithmic, which is needed to properly display a power-law and is referred to as a “log-log” plot. All of the display controls had to be adjusted very carefully for these measurements, especially the black-level. This was done in exactly the same way as described in Part I. Figure 1 shows the Screen Brightness (luminance) in cd/m² (candelas per square meter) for each of the displays as a function of the signal intensity level expressed as a percentage of maximum. To convert to another common luminance unit, foot-Lamberts (fL), divide by 3.43. The open symbols plotted on the graph are the measured data points. (The values for 100 percent are identical to the peak brightness values listed in Part I, with a maximum backlight for the LCD and a 5 percent APL for the Plasma.)

 

You can see that the gray-scales are all reasonably close to straight lines on a log-log graph, but have different slopes. Note how the logarithmic plot emphasizes the dimmest parts of the gray-scale. This parallels the eye’s own extended sensitivity to dark content. (The brightness scale on the graph covers a range of 10,000:1, although the data doesn’t use all of this range. On a linear graph the dimmest parts of the gray-scale would be compressed into a tiny area at the lower left corner, so they would be virtually invisible and the important behavior at the dim-end lost all together.)

 

 

    

 

 

From Figure 1 you can see that the LCD has the steepest gray-scale and the Plasma the shallowest. This explains why sometimes one flat panel display appears the brightest and at other times a different one does. Above 55% signal intensity the LCD is the brightest and the Plasma the dimmest, but below 20% signal intensity the Plasma is the brightest and the LCD the dimmest. Between 20% and 55% the DLP is the brightest, so each of the flat panel displays gets a turn at being number one. At 25% signal intensity all of the flat panels are about equal in brightness. So the relative brightness between the flat panels actually depends on the image content.

 

 

 

 

Figure 2 shows the same plot as Figure 1, only now the peak brightnesses are all equalized to a common 100 percent. This allows us to compare the relative behavior of the gray-scales directly because they now all have the same ratios compared to peak brightness. It’s now easy to see why the displays look different. There is a considerable variation in the steepness of the gray-scales and the differences diverge significantly at lower signal intensities. At 20% signal intensity there is almost a 3:1 difference in the relative brightness between the Plasma and LCD displays. (You would never learn this from a linear graph, where the differences between the displays would appear to be greatest at around 50% signal intensity, which is not correct.)

 

Gamma is the numerical value of the slope (steepness) of the gray-scale when plotted on a logarithmic “log-log” graph. While there are reasons why a Gamma of 3.0 might be considered optimum (based on the eye’s specific power-law response), what is more important is to have a standard Gamma value defined. Television, DVD, Web and computer based photographic content are generally color balanced on professional CRT studio monitors that are electronically adjusted to have a standard Gamma of 2.20, so you’ll get the most accurate images if your display also has this value. Here are the Gammas determined from the log-log plots in the most important region of 100% to 30% signal intensity (which goes down to about 7% of peak brightness in the case of the CRT). Below that the slopes start varying somewhat, which means the Gammas will also vary at the very dark end.

 

 

Gamma Measurements

 

 

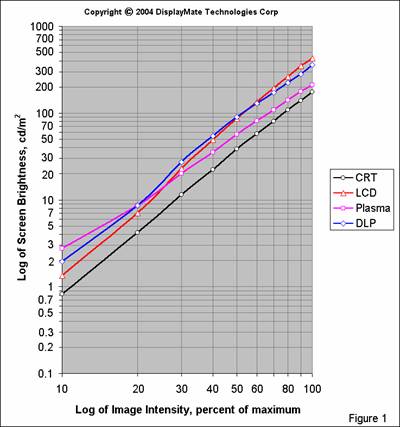
The Gamma for the Sony CRT agrees perfectly with the 2.20 standard value. (CRT monitors from Ikegami, another major brand of professional studio monitors, also have a Gamma of 2.20 according to their Director of Engineering.) The LCD has a Gamma greater than the standard, the DLP is less and the Plasma much less than the 2.20 standard. (Note: the Plasma and DLP displays both provide a choice among several Gamma selections. They are identified only by their menu entry selection numbers, not by their Gamma values. We chose the steepest available for each, which provides the closest agreement with the 2.20 standard.) The real question is how much of a difference do these different gray-scales and values of Gamma make and how do they affect the appearance of an image.

 

 

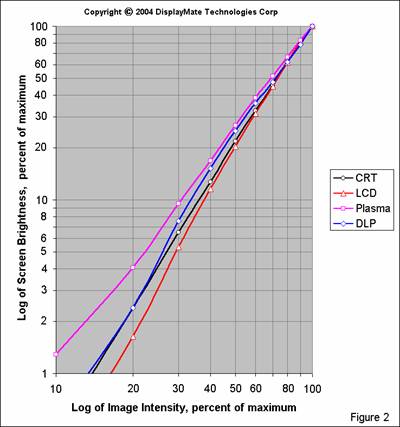
How Gamma Affects the Image

Gamma has a major effect on image brightness, contrast, hue and color saturation. In order to explore this we’ll examine the differences between the LCD, which has the highest Gamma, and the Plasma, which has the lowest. To see this on the graph in Figure 2, compare the relative brightness between the LCD and Plasma at 50% signal intensity and at 25% signal intensity. At 50% intensity the Plasma has a relative brightness that is 1.3 times that of the LCD. At 25% intensity the Plasma has a relative brightness that is 2.1 times that of the LCD.

 

Image Contrast

The higher the Gamma the faster the brightness decreases with signal intensity level. Consider a black and white photograph. The brightness ratio of the bright content to the dark content will be considerably greater on the LCD than on the Plasma. These ratios of brightness are actually just contrast ratios. For example, considering the 25% intensity level, the LCD will have a contrast that is 2.1 times greater than the Plasma. Overall the image on the LCD will appear to have a higher contrast than a standard 2.20 Gamma display and the Plasma will have a lower contrast. Note that this is independent of the Contrast control setting (because it actually affects display brightness and not contrast, see Part I and below) and also independent of the (checkerboard) Display Contrast ratio measured in Part I (as long as the ratio doesn’t fall too low). So Gamma turns out to be the determining factor in visual contrast for images on a display. A control that varies Gamma will function as a true contrast control.

 

Image Brightness

Again, the higher the Gamma the faster the brightness decreases with signal intensity. Since most images have a wide range of intensities, displays with a higher Gamma will appear darker and those with a lower Gamma will appear brighter. Given the industry’s emphasis on brightness, it’s not surprising to find a bias towards lower Gamma values.

 

Hue

When combining the primary colors to produce color mixtures in an image, different Gammas result in different brightness values for the primaries, which produces varying hues and brightness in the resulting colors. For example, when mixing red and green in the ratio of 2 parts red to one part green (100% red intensity and 50% green intensity), which produces a brown, the green will be 1.3 times brighter on the Plasma than on the LCD, so the browns will be different. We verified this visually: the brown was noticeably redder on the LCD than on the Plasma, as expected from their Gammas. From this we see that image hues are significantly affected by Gamma. Gamma will also have a similar effect on flesh tones. While the display’s Tint control can be used to correct the flesh tones to their proper visual appearance, all of the other colors will be modified at the same time, introducing additional errors in hue throughout the image, so a standard Gamma is necessary in order to get accurate color. Most people will tweak the Tint and other controls in order to make the faces come out right. But every tweak that’s used to compensate for a display parameter error leads to a progression of inaccuracies that add up.

 

Color Saturation

Color Saturation is also affected by Gamma in the same way as hue, except that all three primary colors are involved. The primary color with the lowest signal intensity in any color mixture determines the Saturation of the resulting color because it is perceived as combining with equal intensities of the two other primaries to produce a low intensity shade of white (dark gray). This washes out the appearance of the color mixture into a lower saturation pastel. Since Gamma has the greatest effect on the dimmest primary color the brightness of this white (gray) component varies significantly. For example, 75% red, 50% green and 25% blue is perceived as a red-green mixture having a 25% white (gray) component. This white component will be 2.1 times brighter on the Plasma than on the LCD, so the color will have a much lower saturation on the Plasma than on the LCD. As a result color Saturation is significantly affected by Gamma. Higher Gammas increase color saturation and lower Gammas decrease color saturation. The display’s color Saturation control can be used to reduce the saturation error resulting from a non-standard Gamma, but it can’t eliminate it because the saturation error varies with signal intensity. Again, people will tweak the Saturation Control to make the faces come out right, but all of the other colors will be modified at the same time. For a Gamma that’s too low the Saturation Control will need to be turned up and as a result many colors may wind up appearing too saturated. So a standard Gamma is necessary in order to get accurate color saturation at all intensities. Note that a Gamma higher than the standard 2.20 can be used with flat panel displays to counteract the reduced color saturation that’s due to an elevated black-level luminance (because the glow washes out the colors), so it is possible to manipulate this effect into a corrective action.

 

Variations in Gamma

In principle, the gray-scales should appear as perfectly straight lines (power-laws) in Figures 1 and 2. Otherwise the Gamma for a display will vary with signal intensity, and then so will all of the characteristics discussed above, including contrast, hue and saturation. In the brown example above, a display will produce different browns at different signal intensities if the gray-scale deviates from a straight line in a log-log plot. Note that the gray-scale for the reference standard CRT is almost perfectly straight, while there are larger variations for each of the flat panels..

 

Another very important reason why the gray-scales should have a constant Gamma is that if the user adjusts the display’s Contrast Control (which varies the peak brightness, not the contrast) it will shift the portion of gray-scale that is being utilized (the intensities will all shift to the left or right on the graph). If the Gamma isn’t constant the Contrast Control will not only change the peak brightness, but will also introduce unwanted and undesirable changes in image contrast, hue and color saturation.

 

Effect of the Black-Level

Adjusting the black-level can change the shape of the gray-scale at low intensities. If the black-level is raised then the gray-scale will fall less steeply at the dim-end. Conversely, if it’s lowered then the gray-scale will fall more steeply at the dim-end. So if you set the black-level incorrectly, it will change the display’s gray-scale and Gamma. That’s why a Black-Level control is so important. You can also intentionally misadjust the black-level to modify the behavior at the dim-end of the gray-scale. For example, reducing the black-level for the Plasma and increasing it for the LCD would help straighten out their gray-scales at the dim-end. (Note: the black-levels were set very accurately for the measurements in Figures 1 and 2, so the gray-scales are accurate as shown.)

 

Cinema Modes

Many displays and projectors include special “cinema modes” that bring out dark image detail. What they’re actually doing is stretching and artificially raising the lower end of the gray-scale. This behavior is similar to the “base boost” control in audio systems, which intentionally reduces accuracy for crowd pleasing effects. We’ve shown that such effects introduce hue and color saturation errors in addition to affecting brightness and contrast. Gamma controls (when provided) often behave in the same fashion. Rather than actually changing the logarithmic slope of the gray-scale they simply stretch the lower portion of it. In Part III we’ll discuss why stretching the gray-scale also introduces image artifacts.

 

Gamma Control

While we have stressed the importance of having a standard Gamma of 2.20, every display should have a Gamma Control in order to allow image contrast to be adjusted based on variations in the source material, ambient lighting and individual preferences. (Remember that the control labeled “Contrast” actually controls image brightness and does not affect image contrast. See Part I and below.) Also, some source material may be balanced on displays with a non-standard Gamma or possibly just not properly balanced at all.

 

In order to properly adjust image contrast the Gamma control must vary the logarithmic slope of the gray-scale. If the nominal value is 2.20 then a range of plus or minus 0.4 (with steps no greater than 0.1) should be sufficient to accommodate most source material variations and individual preferences. The lower Gamma values are good for improving source material that has too much contrast, or for boosting overall image brightness. The higher Gamma values are good for boosting source material that has insufficient contrast or for improving image contrast that has been reduced due to bright ambient lighting washing out the screen.

 

Following the “base boost” analogy mentioned above we also need to allow for an extra boost in image contrast, which some people prefer. Higher contrast values may also be needed in very dark ambient lighting conditions because the eye tends to reduce visual contrast in those situations (this is known as the Surround Effect). So the Gamma range needs to be extended by an additional 0.4 (on the plus side only) in order to accommodate these effects.

 

Overall a Gamma range of 1.8 to 3.0 should cover just about all situations. For flat panels the Gamma control is easily implemented with digital lookup tables (that can be recalculated real-time), however it’s a lot harder for CRTs if it’s done with analog electronics. The control should be conveniently accessible so that it can be adjusted while viewing an image or test pattern. Note that the hue and saturation errors discussed above that depend on Gamma will still apply unless those effects are corrected through advanced digital signal processing (see below).

 

Gamma Interpretation

There are many reasons why displays have different Gammas. While each technology has its own native Transfer Characteristic or Gamma, the display’s signal processing electronics modifies it in order to obtain the desired gray-scale as accurately as possible. In particular, current CRTs typically have a native Gamma in the range of 2.3 to 2.6, so the Gamma of 2.20 for Sony (and Ikegami) CRT studio monitors is actually the result of signal processing. (Note that the CRT’s power-law behavior originates within its electron gun and has nothing to do with the phosphors.) DLP and Plasma displays have a native Gamma of 1.0 and LCDs have a variable native Gamma that results from an “S” shaped Transfer Characteristic. So signal processing plays an important role in generating a display’s gray-scale.

 

As a result the specific Gamma values that we’ve measured here apply only to these particular models and are not inherent to their particular technologies. However, the behaviors that we have seen here are not arbitrary or accidental, in fact they have been carefully chosen by their manufacturers to compliment each display technology’s strengths and weaknesses. For example, the LCD is currently optimized for computer applications, where signal intensities are frequently near peak. The steep gray-scale produces bright, high contrast images with high color saturation. The Plasma is optimized for video applications, which have much lower signal intensities. The less steep gray-scale helps it deliver a brighter image. The DLP is relatively bright, so it can afford to use a steeper gray-scale at low intensities to enhance visual contrast and color saturation near its black-level. This also cuts down on the visibility of dithering noise in the image. We’ll discuss these issues further in Part III.

 

The Functional Names of User Controls

In Parts I and II we have discussed the functionality and confusing names given to display controls. Below is a summary.

 

Brightness Control:

It doesn’t control brightness, it actually controls the display’s black-level. Its true functional name is: Black-Level Control. Note: on many LCD displays the Brightness Control does instead control the intensity of the backlight, so its name is actually functionally correct there. This variation, of course, adds to the overall level of control confusion.

 

Contrast Control:

It doesn’t control the display’s contrast because it proportionally increases or decreases the entire gray-scale, so none of the brightness ratios change. Technically it varies the video gain. It actually controls the display’s overall brightness. Its true functional name is: Brightness Control.

 

Gamma Control:

If it really controls the Gamma, which is the logarithmic slope of the gray-scale, the functional name for this control is: Contrast Control. Every display should have one in order to allow the image to be properly adjusted. Control name confusion is one reason why almost all displays and projectors are missing this essential control - most people think they already have a Contrast Control due to the mislabeling of the functional Brightness Control.

 

After 75 years of misuse it’s not too likely that this will be straightened out any time soon, but we thought you might just want to know how things should have been named.

 

 

The Primary Chromaticities

The color coordinates of the red, green and blue primary colors in each display defines the gamut of colors that it can reproduce. All of the colors that the display produces are combinations of the primary colors that it uses. In principle, the wider the color gamut the better, and many manufacturers boast about their larger color gamuts. However, variations in the primaries also change all of the displayed colors in an image. So, in practice, it’s generally much more important to use standard primaries in order to increase the color accuracy of reproduced images. Wider color gamuts decrease color accuracy and should be avoided except in specialized applications. This is a perfect example of how more actually turns out to be less.

 

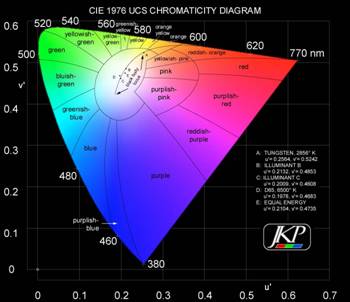
Colors are measured in chromaticity coordinates. Most discussions generally show the 1931 CIE Diagram with x,y coordinates, but the relative distribution of colors is not perceptually uniform for the eye. In particular, it stretches and over emphasizes the eye’s resolution of greens and compresses the reds and blues. In 1976 a Uniform Chromaticity Scale (UCS) with u',v' coordinates was developed in order to provide a perceptually uniform color space. It provides a much more accurate rendering of the eye’s sensitivity to different colors. Equal distances anywhere on the UCS diagram correspond to equally perceived color differences. Figure 3 shows the distribution of colors in this space (figure courtesy of Joe Kane Productions). Note that this figure is only schematic because displays, projectors, film and print media are unable to produce the most saturated colors in the outermost regions of the UCS “horseshoe,” so they wind up being rendered the same as colors in the inner parts of the diagram.

 

 

 

 

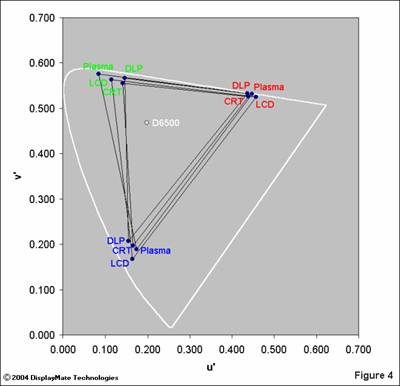
We measured the chromaticity coordinates for the red, green and blue primaries on each display with the Konica Minolta CS-1000 Spectroradiometer and a set of DisplayMate for Windows test patterns. These are shown in a UCS diagram in Figure 4 below, but without including the interior colors for clarity. The outer white line defines the pure spectral colors (upper horseshoe) and the purples connecting the extreme ends of the visible spectrum (lower diagonal line). The CRTs and Plasmas generate their primary colors using light emitting phosphors, while the LCDs and DLPs use color filters together with a light source. Some Plasmas (including the NEC 61XM2) also have filters in order to fine-tune their primary colors.

 

 

 

 

Color Gamut

The color gamut of each display is the area inside the triangle formed by connecting its primary colors. The bigger the triangle the wider the color gamut. Note that directions parallel to the outer white line are differences in hue and directions perpendicular to it are differences in color saturation. The high saturation colors that lie outside of a triangle cannot be reproduced by the display. Instead it generates the closest color that it is capable of producing. This actually isn’t as serious a problem as you might expect because highly saturated colors are seldom found in nature, so a display seldom needs to generate such colors.

 

From Figure 4 we see that the Plasma has the widest color gamut and the DLP the smallest, although of all of the flat panels it has primaries closest to the CRT, which is our reference standard. All of the primaries have a relatively high color saturation because they are close to the outer white border. The major difference between the displays is in the hues of the primaries (rather than their saturation) and the biggest variation is in the greens.

 

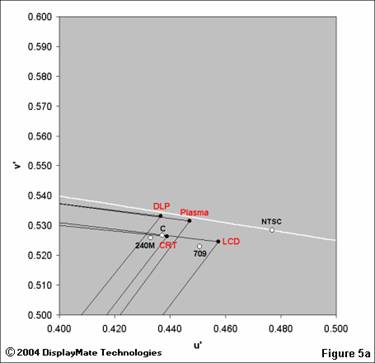
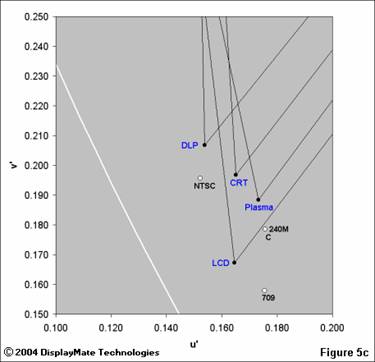
Primary Color Standards

In imaging applications the accuracy of color reproduction is generally what matters the most. That’s why standards for the primary colors are very important. Not surprisingly, many different standards have evolved over the years. They include the original NTSC colors defined in 1953 (now considered obsolete), SMPTE C, SMPTE 240M and ITU-R BT.709 standards. In order to show both the displays and standards together we’ve made separate enlarged Figures 5abc for each primary color. Note that in these figures SMPTE C is shown simply as “C.” Television, DVD, Web and computer based photographic content are generally color balanced on professional CRT studio monitors that have phosphors relatively close to SMPTE C, such as the Sony monitor in this article (Ikegami studio monitors also have SMPTE C phosphors), so you’ll get the most accurate images if your display has these values also. ITU-R BT.709 is actually the official standard for High Definition television, but SMPTE C will continue to reign as the true operational standard until a new generation of monitors with “709” phosphors are introduced and the current generation of studio monitors are replaced. Again, directions parallel to the outer white line are differences in hue and directions perpendicular to it are differences in color saturation.

 

 

 

 

 

It was relatively easy to visually identify the differences between the various primaries using test patterns, photographs and DVDs. They were consistent with the spectroradiometer measurements. The differences are most apparent in images that include highly saturated colors. For red, the DLP and LCD are separated the most. For green the Plasma produced a green that was significantly different than all of the other displays, and is actually quite close to NTSC green. This will have a tendency to add a green caste that can be partially corrected using the display’s color controls (see below). For blue the DLP and LCD are again separated the most. In each case the CRT primaries were in the middle of the pack, which is not surprising given that it is the reference standard so none of the displays can stray too far from it. Overall, the DLP had primaries closest to the CRT, with the Plasma coming in second. (It would have been a tie had the Plasma green not been so far off.)

 

Transforming Primaries

The different sets of primaries produce different color renderings of any image. This results in hue and saturation errors if your display has primaries which are different from the standard that was used to color balance the source material. While it’s possible for any display to electronically transform its primaries into closer agreement with any of the standards, only the professional Sony CRT monitor provided this capability; none of the flat panel displays did so. The electrically transformed primaries are created by adding small percentages of the signal for each primary color to the signals of the other two primary colors. This creates new effective primaries that have altered positions on the chromaticity diagram. Note that transforming primaries can only reduce the display’s native color gamut to a standard gamut, but cannot increase it. If there are regions of the primary color triangle for the standard primaries that lie outside of the color triangle for the native primaries of the display, then colors in those regions will still not be reproduced accurately. Because SMPTE C will eventually be superceded by ITU-R BT.709, displays should be able to switch between either standard, depending on the source material being viewed. In the absence of this capability, the Tint and Saturation controls can be used to get the most critical colors correct, generally the flesh tones, but that will again introduce additional color errors throughout the image.

 

 

 

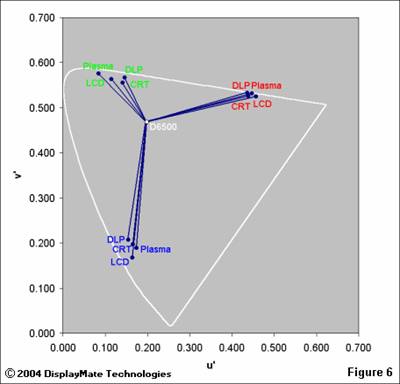
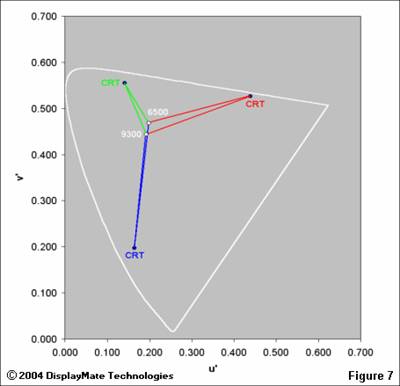
Primary and White-Point Variations

Another way to illustrate the variations in primaries is shown in Figure 6, which connects the D6500 white-point to all of the primaries. These vectors show the different color tracks for each of the primaries as the saturation goes from zero at the D6500 white-point to a fully saturated primary color. The reds are tightly clustered because they play a critical role in reproducing pink flesh tones. Larger variations, like those for the greens, would require large adjustments for the Tint Control in order to correct the resulting variation in flesh tones, and that would throw off all of the other colors significantly. Figure 7 shows how a change in the white-point from D6500 to 9300 K affects the primary color tracks for the CRT. (The other display technologies are not shown for clarity.) The blue tracks closely overlap, indicating that the change is equivalent to a shift in blue saturation. The biggest difference is in the red tracks, where there are both hue and saturation shifts. Note that the location of the white-point has the greatest effect on low saturation colors and the least for high saturation colors. One way to produce better flesh tones from a 9300 K white-point is to enhance the red signal so that it shadows the 6500 K track more closely. This is the origin of “red push,” which is generally found in video displays set up for 9300 K at the factory.

 

 

 

The Holy Grail of Color and Gray-Scale Accuracy

The signals that are delivered to a display or projector and then subsequently processed by its internal electronics are currently handled as a set of electronic signals, generally in the form of native RGB, or encoded, such as YP_BP_R component or composite video. They are processed as parameterized signals and not as luminance and chromaticity values. The display electronics transforms them from one signal form into another as needed in order to accomplish its processing tasks, such as implementing the user, service and calibration controls and preparing the signals for the actual display device. This method introduces color and gray-scale errors because the processing takes place in signal space rather than color space.

 

A much better method would be to first transform those signals (real-time pixel by pixel) into luminance and chromaticity coordinates L,u',v', process them in this form, and then finally transform them back into electronic signals for final delivery to the display device. This is computationally more difficult and has not yet been implemented.

 

There are major advantages to this approach: it separates the calibration and parameter settings of the source material from the calibration and parameter settings of your display or projector; it eliminates the Gamma, hue and color saturation interactions and errors that we have discussed; it improves the functionality and accuracy of the user, service and calibration controls; and best of all it can produce absolute color and gray-scale accuracy from a wide variety of source material while also allowing variations based on user preferences. Another big plus is that it will be possible to accurately correct the photometric and colorimetric errors introduced by the black-level luminance glow that is produced by all non-CRT technologies. It won’t lower or get rid of the glow but it will eliminate the color and gray-scale errors that it causes above the black-level.

 

What’s involved: first you must know or assume (see below) the calibration and parameters of the monitor that were used to balance the source material (the Gamma, primary and white-point chromaticities – for example 2.20, SMPTE C and D6500) and select them from a Source Menu. This allows L,u',v' to be calculated from the input signals. User controls like Gamma, color Saturation and Tint can then be functionally and accurately implemented. For example, varying the display’s visual contrast with Gamma will no longer introduce hue and saturation errors. Once the processing is completed the electronic signals can be accurately regenerated based on the display’s (known) native parameters like the Primary Chromaticities and Transfer Characteristic, which are set at the factory. Field calibration will involve remeasuring these parameters, particularly as the display ages. (It will no longer be necessary to set the white-point chromaticity or color temperature of the display because this method will automatically reproduce whatever value was used in the source material.)

 

Instead of playing with the Tint, Saturation, Black-Level and other controls to get the picture looking right, you’ll choose from a number of Source Menu selections. For source material that has been professionally balanced there are only a small number of choices, even if you initially don’t know which one was used. So the right choice should be reasonably easy to visually identify if your display is properly calibrated. (The parameters could be encoded into the video stream, which would make the entire process automatic.) The end result will be photometrically and colorimetrically accurate images. Functionally accurate controls like Gamma, color Saturation and Tint will still be available to accommodate individual preferences. All of the current ad-hoc controls, signal processing, and user tweaking that often leads to images with murky fidelity will be gone. That’s why we consider this method to be the Holy Grail of color and gray-scale accuracy. While it requires considerably more complex signal processing it is not beyond the capabilities of current electronics and hopefully we’ll see this approach in the near future.

 

 

Closing

In Part II of the article we have examined the many facets involved in achieving gray-scale and color accuracy. In each case the key has been to closely follow basic principles, established standards and the operational behavior of reference displays. Having a large selection of functional controls is essential in calibrating a display, and in correcting or compensating for non-standard (or sub-standard) performance. Still, there is a limit as to what even a multitude of controls can accomplish. Since many display parameters are interdependent, in most cases the controls can only partially compensate for inaccuracies, generally minimizing one inaccuracy at the expense of another. The effects of adjusting various controls are also interdependent, so the result is often a complex dance of pushing and pulling among several interacting controls. You’ll generally discover a number of different “optimum” settings, with no clear sign as to which one is the best or correct one. The complex interaction of so many parameters and controls is why carefully designed test patterns are needed for display set up and calibration. It’s almost impossible to calibrate a display using a photographic image because the image is itself very complex and its makeup emphasizes a particular mix of (unknown) parameters. So when you tweak the controls to optimize that particular image it’s quite likely that later images will call for their own tweaks. In the end, the best approach is to start off with a display that follows all of the standards as closely as possible and then objectively calibrate it with the appropriate suite of test patterns.

 

 

What’s Coming Next

In Part III we’ll examine the complex world of display artifacts for each of the display technologies and in Part IV we’ll analyze and assess each of the display technologies in detail and tie together all of the results from Parts I to IV.

 

 

Acknowledgements

Special thanks to Dr. Edward F. Kelley of the NIST, National Institute of Standards and Technology, for many interesting discussions and for generously sharing his expertise, to Craig Verbeck of Pixelworks for many interesting discussions on signal processing, and to Alan Keil of Ikegami Electronics for technical information on Ikegami monitors. Special thanks to the Konica Minolta Instrument Systems Division for providing editorial loaner instruments whenever and wherever they have been needed and for providing the CS-1000 Spectroradiometer on a long-term loan for this project.

 

 

About the Author

Dr. Raymond Soneira is President of DisplayMate Technologies Corporation of Amherst, New Hampshire. He is a research scientist with a career that spans physics, computer science, and television system design. Dr. Soneira obtained his Ph.D. in Theoretical Physics from Princeton University, spent 5 years as a Long-Term Member of the world famous Institute for Advanced Study in Princeton, another 5 years as a Principal Investigator in the Computer Systems Research Laboratory at AT&T Bell Laboratories, and has also designed, tested, and installed color television broadcast equipment for the CBS Television Network Engineering and Development Department. He has authored over 35 research articles in scientific journals in physics and computer science, including Scientific American. If you have any comments or questions about the article, you can contact him at dtso@displaymate.com.

 

 

Article Links

Series Overview

Part I: The Primary Specs

Part II: Gray-Scale and Color Accuracy

Part III: Display Artifacts and Image Quality

Part IV: Display Technology Assessments

 

 

Copyright © 1990-2005 by DisplayMate Technologies Corporation. All Rights Reserved.
This article, or any part thereof, may not be copied, reproduced, mirrored, distributed or incorporated
into any other work without the prior written permission of DisplayMate Technologies Corporation